Rules - note that means a non-zero digit 123456789 1. All the numbers in the measurement are significant.
Notes 3 1 Part 2 Significant Figures Significant
Did you know that now the speed of light is also an exact number.
Exact number sig figs example. Digits 1 to 9 always count 2. Sum the following three measurements and report to the correct sig figs. An exact number is a value that is known with complete certainty.
2040 - 3 sig fig 2. 1 inch is defined as 254 cm therefore it this is an exact conversion factor. The Sig Fig Rules for ComputationAddition andor Subtraction The answer cannot be more precise than the least precise measurement.
Exact numbers have an unlimited number of sig figs. Exact numbers can be considered as having an unlimited number of significant figures. 00500 x 104 has three.
Example 3201 m 5325 m 12 m Added together. The fewest number of sig figs used in the calculation. In such numbers all of the digits are significant.
Significant figures of a number in positional notation are digits in the number that are reliable and absolutely necessary to indicate the quantity of something. The number of employees in an office will always be 15 or 56 or 70. Your final answer is therefore limited to three sig figs.
Exact numbers such as the number of people in a room have an infinite number of significant figures. It has been determined that exactly 60 seconds are in a minute so 60 has an unlimited number of sig figs. Digits other than zero are always significant.
Infinite precision measurements are an impossibility It does not apply to integers declared conversion constants. Another example of this are defined numbers such as 1 foot 12 inches. That is literally the meaning of exact Significant figures apply only to measurements known to have some accuracy bounds as a means of not overstating their precision.
Zeros after a do not count unless they are also after a decimal place 4. Because it is a measurement the number has significant figures. For example if we want to calculate how many ounces are in 20 lb we would set up the problem thus.
The answer should have three significant figures. The significance of trailing zeros in a number not containing a decimal point can be ambiguous. 412945 has 6 sig figs.
In the example below the quantity with the fewest number of sig figs is 272 three sig figs. When multiplication and division are involved in a series of calculations the final answer must have as many sig figs as the measurement with the least number of sig figs. A class may contain exactly 25 students.
The answer has 3 sig. Fig and not by 16 because it is an exact number. It is the number of digits not the number of sig figs in the mantissa 7.
000018 would have only two significant figures1 and 8. For example there are exactly 3 feet in 1 yard. Number of significant figures in the final calculated value will be the same as that of the quantity with the fewest number of sig figs used in the calculation.
For example the value of 1000 millimeters in a meter has 4 significant digits although it contradicts the sig fig rules. Examples of exact numbers are counted numbers of objects or certain unit conversions. I think this example shows everything I never understood about sig figs.
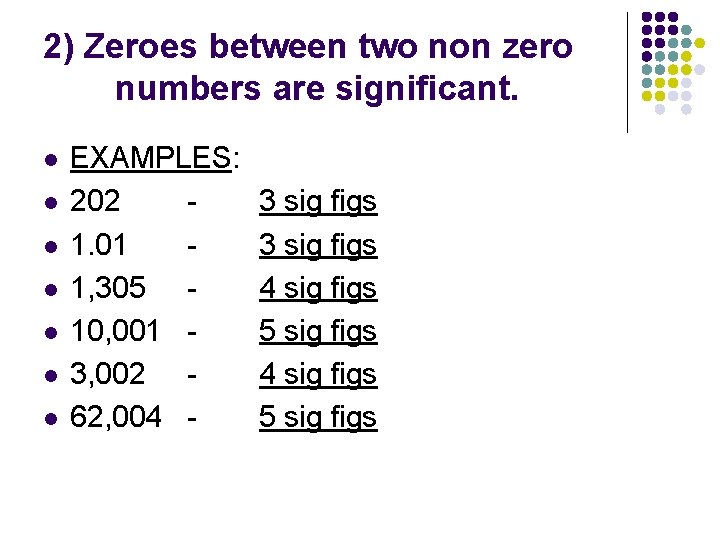
What exact numbers are used in chemistry. Zeros in between any digits that count count also B. For example 10123 17 or 17 x 101.
There are exactly 12 inches in one foot. 00250 has two significant figures. Exact numbers are counting up how many of something are present they are not measurements made.
If a number expressing the result of measurement of something has more digits than the digits allowed by the measurement resolution only the digits allowed by the measurement resolution are reliable and so only these can be significant. There are exactly 12 eggs in a dozen. The measurement 275 kg has three 3 significant figures.
2 All exact numbers have an unlimited number of sig figs. Exact numbers such as the number of people in a room have an infinite number of significant figures. Experts depicted that numbers that have complete certainty are indicated as exact numbers Remember that theres no possibility of uncertainty in exact numbers.
Such values are called exact numbers. It has been determined that exactly 60 seconds are in a minute so 60 has an unlimited number of sig figs. If you counted the number of people in your class to be exactly 35 then 35 would have an unlimited number of sig figs.
Digits because they are exact numbers or definitions. 2 All exact numbers have an unlimited number of sig figs. 258 26 2575 ----- The simple sum is 7755.
However in the measurement. One number goes to the tenths place. So what is an exact number and how many sig figs does this number have.
Sometimes numbers used in a calculation are exact rather than approximate. This has 2 sig figs because there are 2 digits in the mantissa 23. These values come from actual counting and not from experiments.
If you counted the number of people in your class to be exactly 35 then 35 would have an unlimited number of sig figs. Exact numbers are counting up how many of something are present they are not measurements made with instruments. 13 pi4122 147034 The 130 height could really be anywhere from 1295 to 1305.
The answer is limited by 200 lb 3 sig. For example 1 meter 100 meters 10000 meters 10000000000000000000 meters etc. Since 50 51 52 153 and 153 following the sigfig rules for addition produces a number with three sig-figs has three significant figures the answer should also have three significant figures.
Trailing zeros in a number containing a decimal point are significant. And the 120 diameter could be 1195 to 1205. For exponents the number of sig figs is the same as the number of digits in the mantissa.
Therefore if a number is exact it. This rule applies to numbers that are definitions. You measure the mass of an object to be 275 kg.
The 3 is an exact number so it does not limit the number of significant figures contained in the result. Exact numbers have an INFINITE number of significant figures. Leading zeros are not significant.
Final zeros after a decimal point are always significant. Zeros in front never count 3. In practice find the quantity with the fewest number of sig figs.
412945 has 6 sig figs. Solve by using decimal place. The mantissa is 021 and has 3 digits because 105 has 3 sig figs.
If you just multiply it out what do you get. An alternate view point. Even though 16 appears as 2 sig.
3 3 Significant Figures And Rounding Off Chemistry Libretexts
Significant Figures Sig Figs Sig Figs Scientists Use